The Mandelbrot Set: Mandelbrot Set And Chaos Theory

The Mandelbrot set is a fascinating mathematical object that reveals intricate beauty and complex behavior within the realm of chaos theory. It’s like a hidden world, full of surprises and patterns that emerge from simple rules. Imagine zooming into a tiny spot on the set, and you’ll find an entirely new landscape, filled with even more detail. It’s like discovering a whole new universe within a single point!
The Mathematical Formula
The Mandelbrot set is defined by a simple yet powerful formula. It involves repeatedly applying a specific calculation to a complex number. The formula is:
zn+1 = zn2 + c
Here, ‘c’ represents a complex number, and ‘z’ is a variable that starts at 0. We repeatedly apply this formula, substituting the result of each iteration back into the formula. If the magnitude of ‘z’ stays bounded (meaning it doesn’t grow infinitely large), then the complex number ‘c’ belongs to the Mandelbrot set.
Generating the Mandelbrot Set, Mandelbrot set and chaos theory
To generate the Mandelbrot set, we use a process called iteration. It’s like playing a game of “what if” with numbers. We start with a complex number ‘c’ and repeatedly apply the formula, keeping track of the magnitude of ‘z’. If ‘z’ stays bounded after a certain number of iterations, we color the point ‘c’ black. If ‘z’ escapes to infinity, we color the point ‘c’ based on how many iterations it took to escape. This process creates a stunning visual representation of the Mandelbrot set, revealing its intricate details and boundaries.
Zoom Levels and Intricate Details
The Mandelbrot set is known for its infinite complexity. As you zoom into the set, you’ll discover an endless array of smaller and smaller details. It’s like a never-ending journey through a fractal landscape. For example, if you zoom into a particular area, you might find a miniature version of the entire Mandelbrot set, complete with its own intricate features. This self-similarity is a key characteristic of fractals. It’s like finding a tiny version of a whole universe within a single point!
Fractals and the Mandelbrot Set
The Mandelbrot set is a prime example of a fractal. Fractals are geometric shapes that exhibit self-similarity at all scales. This means that if you zoom in on a part of a fractal, you’ll find a smaller version of the original shape. The Mandelbrot set’s infinite complexity arises from its fractal nature. It’s like a never-ending story, with new details unfolding at every level of magnification.
Exploring Chaos Theory

Chaos theory is a branch of mathematics that studies complex systems that are highly sensitive to initial conditions. This sensitivity is often referred to as the “butterfly effect,” where a small change in one part of the system can lead to large and unpredictable changes in the overall behavior of the system.
Sensitivity to Initial Conditions
The butterfly effect is a core concept in chaos theory. It highlights the fact that even tiny differences in starting conditions can lead to vastly different outcomes in chaotic systems. This sensitivity makes it incredibly difficult to predict the long-term behavior of chaotic systems, as even the smallest error in measuring initial conditions can result in significant deviations in the system’s evolution.
Examples of Chaotic Systems in Nature
Chaotic systems are ubiquitous in nature, from the weather to the flow of water. For example, weather patterns are inherently chaotic, making long-range weather forecasting challenging. Even a slight change in atmospheric conditions, like a butterfly flapping its wings, can cascade into a series of events that ultimately affect the weather in distant locations.
Similarly, the flow of water in rivers and streams is chaotic, influenced by factors like the shape of the riverbed, the velocity of the water, and the presence of obstacles. These factors can interact in complex ways, making it difficult to predict the precise path of water flow.
Role of Feedback Loops and Nonlinearity
Feedback loops and nonlinearity play crucial roles in shaping the behavior of chaotic systems. Feedback loops occur when the output of a system influences its own input, creating a cycle of interactions. For instance, in weather systems, rising air temperatures can lead to increased evaporation, which can further increase air temperatures, creating a positive feedback loop.
Nonlinearity refers to the fact that the relationship between the input and output of a chaotic system is not always proportional. Small changes in input can lead to disproportionately large changes in output, contributing to the unpredictability of chaotic systems.
Deterministic Chaos vs. Random Processes
Deterministic chaos refers to systems that are governed by deterministic rules, meaning that their behavior is determined by their initial conditions and the rules that govern their evolution. However, despite being deterministic, these systems can exhibit chaotic behavior due to their sensitivity to initial conditions.
Random processes, on the other hand, are inherently unpredictable and do not follow any specific rules. Their behavior is governed by chance or randomness.
While deterministic chaos and random processes are distinct concepts, they can sometimes be difficult to distinguish in practice. This is because the chaotic behavior of deterministic systems can appear random, especially when observed over long periods.
The Connection Between Chaos and the Mandelbrot Set
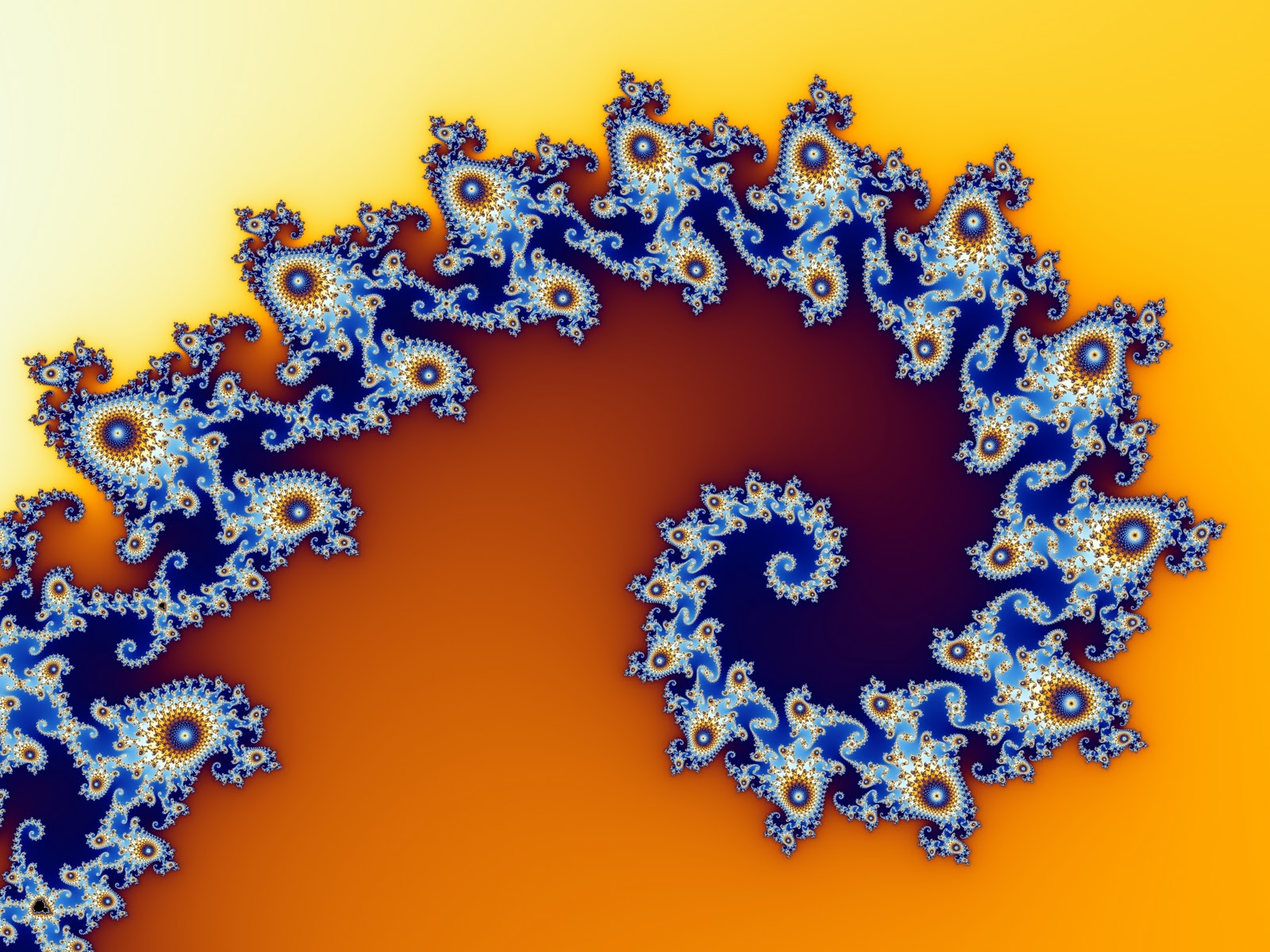
The Mandelbrot set is a fascinating mathematical object that perfectly illustrates the principles of chaos theory. It’s like a window into a world where seemingly random patterns emerge from simple rules.
The Mandelbrot set, with its intricate and unpredictable patterns, showcases the core concepts of chaos theory. It’s a visual representation of how small changes in initial conditions can lead to wildly different outcomes.
Self-Similarity Within the Mandelbrot Set
The Mandelbrot set exhibits self-similarity, meaning that smaller parts of the set resemble the whole. Think of a fractal—zoom in on any part, and you’ll find the same intricate patterns repeating.
The Mandelbrot set is a fractal, a shape that repeats itself at different scales.
This self-similarity is a key characteristic of chaotic systems. Imagine looking at a coastline—the jagged edges repeat themselves at different scales, from small inlets to large bays. The Mandelbrot set is like a mathematical coastline, revealing this repeating structure in a mesmerizing way.
The Role of Complex Numbers
The Mandelbrot set is generated using complex numbers, which are numbers that include both real and imaginary parts. Think of them like a special type of number that expands our understanding of math beyond the typical numbers we use every day.
Complex numbers are numbers of the form a + bi, where ‘a’ and ‘b’ are real numbers, and ‘i’ is the imaginary unit, which is defined as the square root of -1.
The Mandelbrot set uses a simple equation involving complex numbers. By iterating this equation, meaning repeating it over and over again, we can determine whether a given complex number belongs to the Mandelbrot set or not.
Chaotic Behavior Within the Mandelbrot Set
The Mandelbrot set is full of chaotic behavior. Imagine zooming in on a specific area of the set—you might find that small changes in the starting point lead to drastically different outcomes.
The Mandelbrot set exhibits chaotic behavior, meaning that small changes in initial conditions can lead to wildly different outcomes.
Think of a weather forecast—even with the most sophisticated models, predicting the weather more than a few days out is difficult because of the chaotic nature of the atmosphere. The Mandelbrot set provides a visual representation of this chaotic behavior, where small changes can create massive differences.
Mandelbrot set and chaos theory – The Mandelbrot set, a captivating fractal, exemplifies the intricate beauty born from chaos theory. Its infinite complexity, arising from simple iterative equations, echoes the delicate patterns found in nature, such as the intricate grain of wood. These natural patterns inspire artisans to craft furniture like the stunning wood designs square table and chair sets , where the inherent beauty of the wood is celebrated.
Just as the Mandelbrot set reveals hidden depths within its seemingly chaotic structure, these furniture pieces showcase the artistry that emerges from the seemingly random nature of wood grain.
The Mandelbrot set, a mesmerizing fractal, exemplifies the beauty of chaos theory. Its intricate patterns, born from simple mathematical equations, echo the unpredictable yet elegant dance of nature. Imagine finding solace amidst this mathematical wonder, nestled in the comfort of a benchmaster swivel recliner chair & ottoman set , a haven of relaxation where the complexities of the world fade away.
Just as the Mandelbrot set reveals hidden order within chaos, the chair’s design offers a sanctuary of comfort, a place to ponder the intricacies of the universe and find tranquility in the midst of it all.
